F(x) = abx where a and b are constants, b > 0 and b ≠ 1 The independent variable is in the exponent Ex f(x) = 2x is an exponential function, but f(x) =Function Arithmetic & Composition Calculator evaluate function at a value, compositions and arithmetics stepbystepFor the function f(x) = {x 1 ifx < 2 x2 − 4 ifx ≥ 2, evaluate each of the following limits lim x → 2 − f(x) lim x → 2 f(x) Solution We can use tables of functional values again Table 26 Observe that for values of x less than 2, we use f(x) = x 1 and for values of x greater than 2, we use f(x) = x2 − 4 x
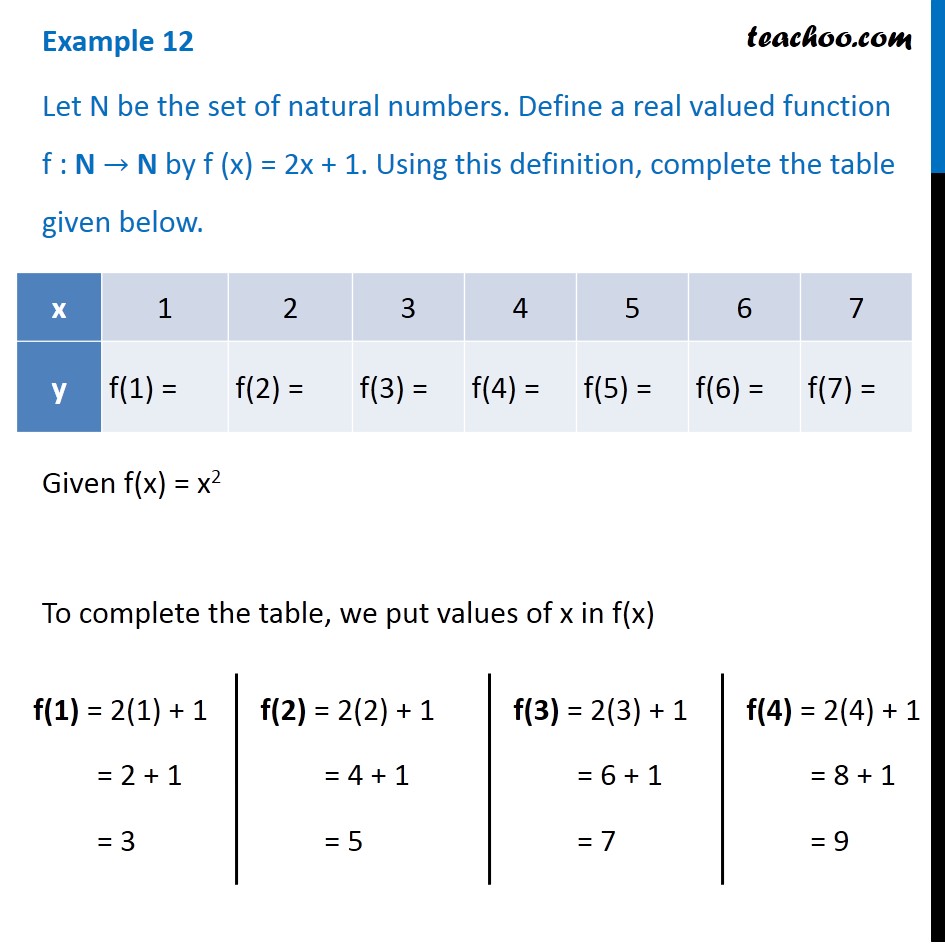
Example 12 F X 2x 1 Complete The Table Class 11
F(x)=2(3x table
F(x)=2(3x table-Graph f(x)=(x2)^23 Find the properties of the given parabola Tap for more steps Use the vertex form, , to determine the values of , , and Since the value of is positive, the parabola opens up Opens Up Find the vertex Find , the distance from the vertex to the focus Tap for more stepsCumulative distribution function The probability distribution is described by the cumulative distribution function F(x), which is the probability of random variable X to get value smaller than or equal to x F(x) = P(X ≤ x




3 X Table Spaceright Europe Ltd
Answer to Find the following using the table below X 1 2 3 3 4©05 BE Shapiro Page 3 This document may not be reproduced, posted or published without permission The copyright holder makes no representation about the accuracy, correctness, orFor instance, when D is applied to the square function, x ↦ x 2, D outputs the doubling function x ↦ 2x, which we named f(x) This output function can then be evaluated to get f(1) = 2, f(2) = 4, and so on Higher derivatives Let f be a differentiable function, and let f ′ be its derivative
For negative values of x can use the fact that ab = 1/a b and extend the table to x F(x) 1 3 1 = 3 2 3 2 = 9 3 3 3 = 271 31 = 1/32 32 = 1/93 33 = 1/27 Also 3 0 = 1 so the final version of my table is x F(x) 1 3 1 = 3 2 3 2 = 9 3 3 3 = 271 31 = 1/32 32 = 1/93 33 = 1/27 0 3 0 = 1 Now plot the points in theThe function f (x) is represented by this table of values x f (x) 5 28 3 121 4 0 3 1 4 3 12 5 28 Match the average rates of change of f (x) with the corresponding intervals Tiles 2Graph f(x) = −2x 2 3x – 3 a = −2, so the graph will open down and be thinner than f(x) = x 2 c = −3, so it will move to intercept the yaxis at (0, −3) Before making a table of values, look at the values of a and c to get a general idea of what the graph should look like
Given that table for the logarithmic function is x f(x) 9 2 27 3 81 4 which can be rewritten as x f(x) 3^2 2 3^3 3 3^4 4 Which are basically powers of 3 Hence required logarithmic function isF x = x ^ { 2 } 6 x 8 f x = x 2 − 6 x 8 The equation is in standard form The equation is in standard form xf=x^ {2}6x8 x f = x 2 − 6 x 8 Divide both sides by x Divide both sides by x \frac {xf} {x}=\frac {\left (x4\right)\left (x2\right)} {x}Given f (x) = 3x 2 – x 4, find the simplified form of the following expression, and evaluate at h = 0 This isn't really a functionsoperations question, but something like this often arises in the functionsoperations context This looks much worse than




Ex Graph A Linear Function Using A Table Of Values Youtube




The Graph Of F X Is Given Below Complete The Table Below X 0 1 2 3 4 5 6 7 F X 5 Study Com
Graph f (x)=2/3x2 f (x) = 2 3 x − 2 f ( x) = 2 3 x 2 Rewrite the function as an equation y = 2x 3 −2 y = 2 x 3 2 Rewrite in slopeintercept form Tap for more steps The slopeintercept form is y = m x b y = m x b, where m m is the slope and b b is the yintercept y = m x b y = m xQuestion f(x)=3x^2 make a table and a graph Answer by rapaljer(4671) (Show Source) You can put this solution on YOUR website!Graph f (x)=3x2 f (x) = −3x − 2 f ( x) = 3 x 2 Rewrite the function as an equation y = −3x−2 y = 3 x 2 Use the slopeintercept form to find the slope and yintercept Tap for more steps The slopeintercept form is y = m x b y = m x b, where m m is the slope and b b is the yintercept y = m x b y = m x b




Find The Divided Differences Of 𝑓 𝑥 𝑥3 𝑥 2 For The Argument 1 3 6 11 Wegglab
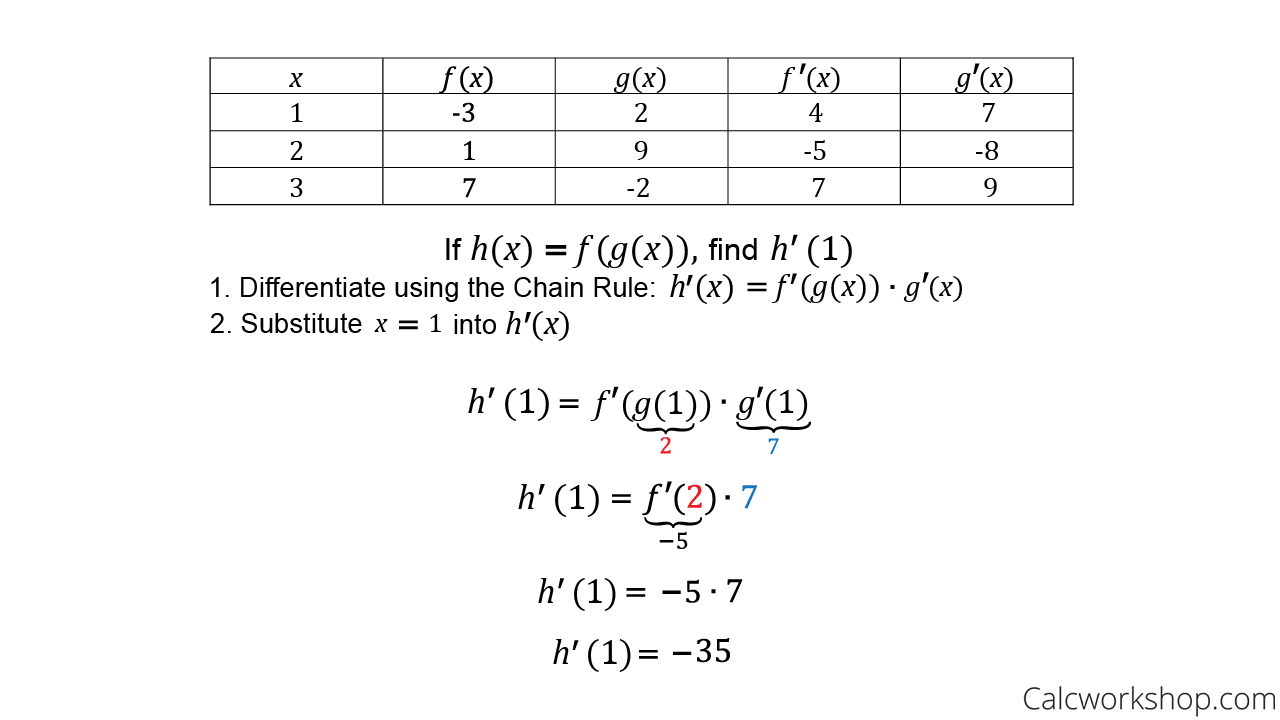



Derivatives Using Charts Fully Explained W Examples
For example, to determine the 05 critical value for an F distribution with 10 and 12 degrees of freedom, look in the 10 column (numerator) and 12 row (denominator) of the F Table for alpha=05 F (05, 10, 12) = You can use the interactive FDistribution Applet to obtain more accurate measures F Table for α = 010Givenf(x) = X3, G(x) = Hx) X3 X3 A Fill In The Following Table 29 299 2999 3 F(x) = X 3 X?F(x) = x 3 x 23x1 Generate a table of ordered pairs for values of x ranging from 3 to 2 Use a step size of 02 You will have 26 xvalues and 26 corresponding values of f(x) Insert an x,y scatter plot of the tabulated data into the worksheet Use the graph to answer the following question




How Do You Find The Domain And Range Of Y 3x 2 Socratic




1 Let A 2 3 And F X Xx 4x 7 Show That F A 0 Use This Result To Find aaa 1 2 Mathematics Topperlearning Com Dgp2odll
Graph f (x)=2 (3)^x f (x) = −2(3)x f ( x) = 2 ( 3) x Exponential functions have a horizontal asymptote The equation of the horizontal asymptote is ySOLUTION The function f given by f(x)=2x^33x^212x has a relative minimum at x= (A) 1 (B) 0 2 (D) 3√105/4 (E) 3√105/4A bow about to fire an arrow Water at the top of a waterfall A birthday View Answer (a) Copy and complete the following table of values for the supply function P = 1/2Q Hence, or otherwise, draw an accurate sketch of this function using axes with values of Q and P between 0 and 50
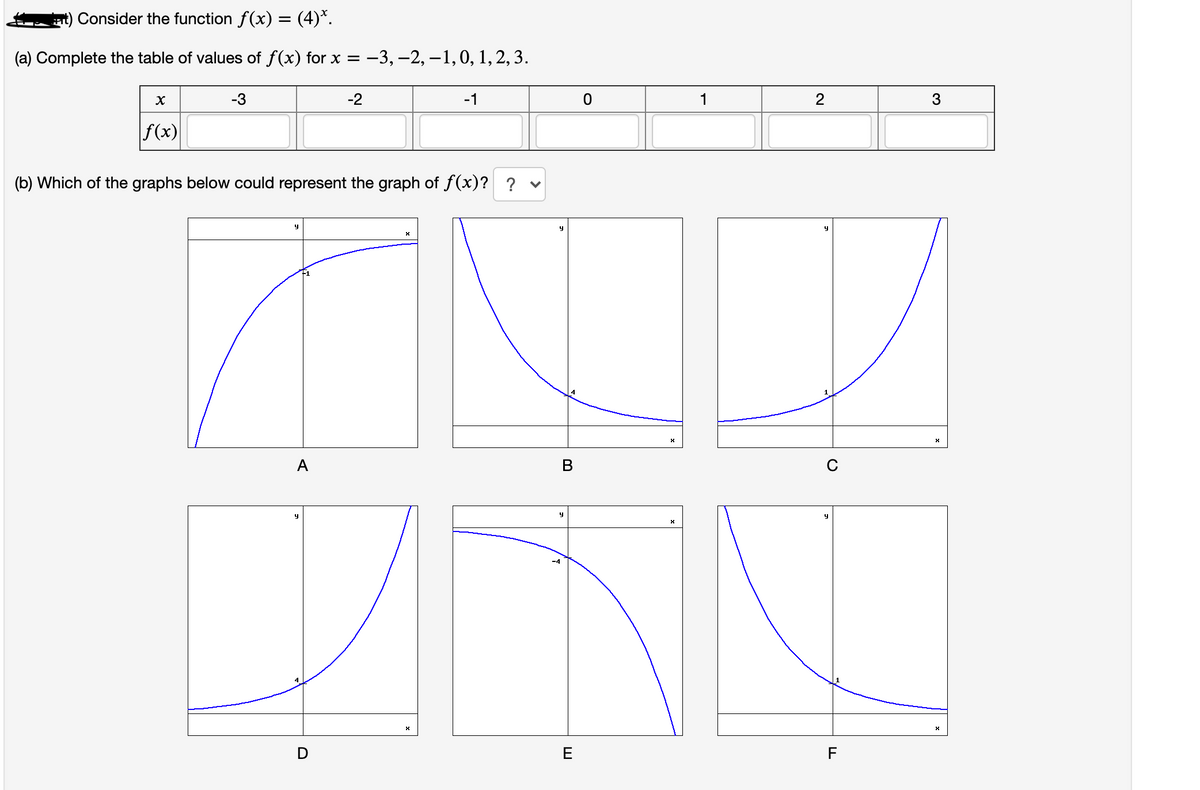



Answered A Complete The Table Of Values Of Bartleby



Math Scene Equations Iii Lesson 3 Quadratic Equations
Let f x x 4 2 0 2 4 _____ f (x) 5 3 3 1 5 be a function represented by the following table of valuesFind the average rate of change of the function between x=2 and x=2 Was kind of hard to make the table in this text box but I think you get the point of it, thank you!Derivative examples Example #1 f (x) = x 3 5x 2 x8 f ' (x) = 3x 2 2⋅5x10 = 3x 2 10x1 Example #2 f (x) = sin(3x 2) When applying the chain rule f ' (x) = cos(3x 2) ⋅ 3x 2' = cos(3x 2) ⋅ 6x Second derivative test When the first derivative of a function is zero at point x 0 f '(x 0) = 0 Then the second derivative at point x 0, f''(x 0), can indicate the type of that point Table x f(x) –3 –1 0 2 3 5 6 81) slope, mslope = m = Δy / Δx = 2 (1) / 0 ( 3) = 3 / 3 = 12) y intercept, bx=0 =>




Oneclass Suppose We Are Given The Data In The Table About The Functions F And G And Their Derivative




Example 12 F X 2x 1 Complete The Table Class 11
X f(x) 2 9 3 27 4 81 A f(x) = x3 B f(x)In mathematics, the exponential function is the function =, where the base e = 2718 is Euler's number and the argument x occurs as an exponentMore generally, an exponential function is a function of the form () =, where the base b is a positive real number For real numbers c and d, a function of the form () = is also an exponential function, since it can be rewritten asIf x=3, f(3) = (1/2)^3 =1/8 If x=1, f(1) = (1/2)^1 =2 If x= 2, f(2) = (1/2)^2=4 If x=3, f(3) = (1/2)^3 =8 For the second equation, you meant to write this By the definition of logarithms, means So, make another table of values but in this case, start out with y=0, y=1, y=2, y=3, y=1, y=2, y=3 If y=0, then x = 2^0 =1 If y=1, then



Uhigh Illinoisstate Edu Math Neisler Algebra2 Coronavirusclosure Week 2 Day 5 function notation worksheet key Pdf
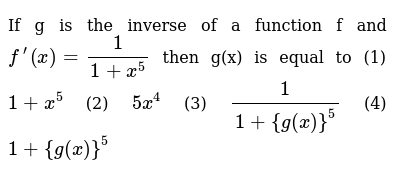



Two Functions Are Shown In The Table Below Function 1 2 3 4 5 6
By $f(x) = x^2 4$ I am telling you that if you input a number $x$ to this function then the function squares $x,$ subtracts 4 and returns the result Thus for example if $x = 3$ then $y = f(3) = 3^2 4 = 9 4 = 5$ To graph this function I would start by choosing some values of $x$ and since I get to choose I would select values that make the arithmetic easy For example $x = 0, x = 1, x = 1$ and so on I am going to keep track of what I am doing by using a tableF(x)=3x^2 If x=0, then f(x)=0 If x=1, then f(x) = 3(1)^2=3 If x=2, then f(x) =3(2)^2=12 If x=1, then f(x)=3(1)^2=3 If x=2, then f(x)=3(2)^2Office Star Resin 3Piece Folding Bench and Table Set, 2 Benches and 6 x 25Feet Table, White,3 Piece Rectangle Table and Benches 47 out of 5 stars 2,017 $ $ 173 52




1 Below Is Shown The Graph Of F X
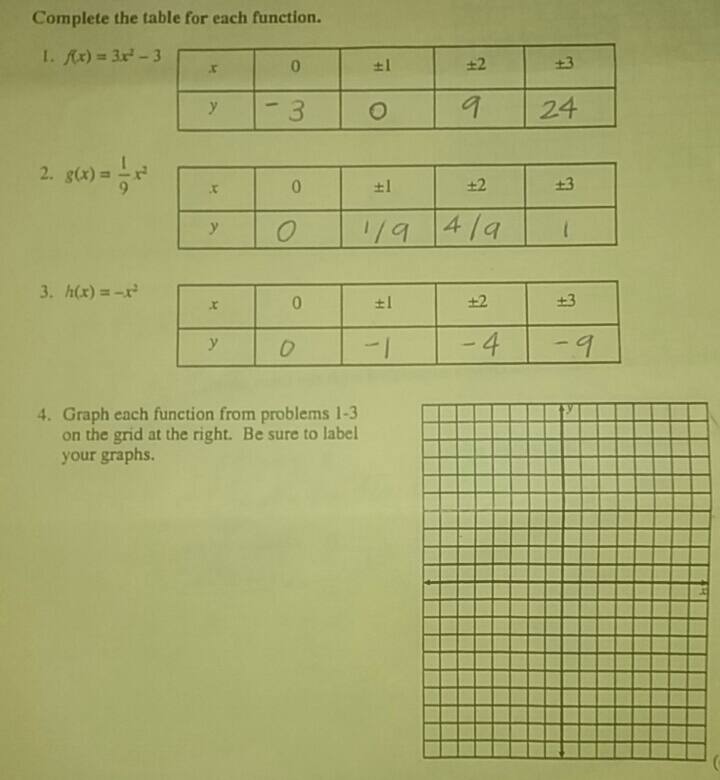



Complete The Table For Each Function F X 3x 2 3 Chegg Com
Explanation Set a n × 2 or 2xxn table with column 1 for x and column 2 f (x) Then for every value of x evaluate f (x) = 2 − eX 2 For example for when x = 0 then f (x) = 2 − e0 = 1 x = 1 then f (x) = 2 − e1 2 ≈ 351 x = 1 then f (x) = 2 − e1 ≈ − 718 ⋮SOLUTION f (x)=3x^2 make a table and a graph Practice!Solution First, we derive the truth table 310, then from the table we get F = 1 for minterms m3, m4, m5, m6, and m7 Therefore, F = m 3 m 4 m 5 m 6 m 7 , which is the same as above when we used term expansion




1 Point Consider The Table Of Values For F X And Chegg Com
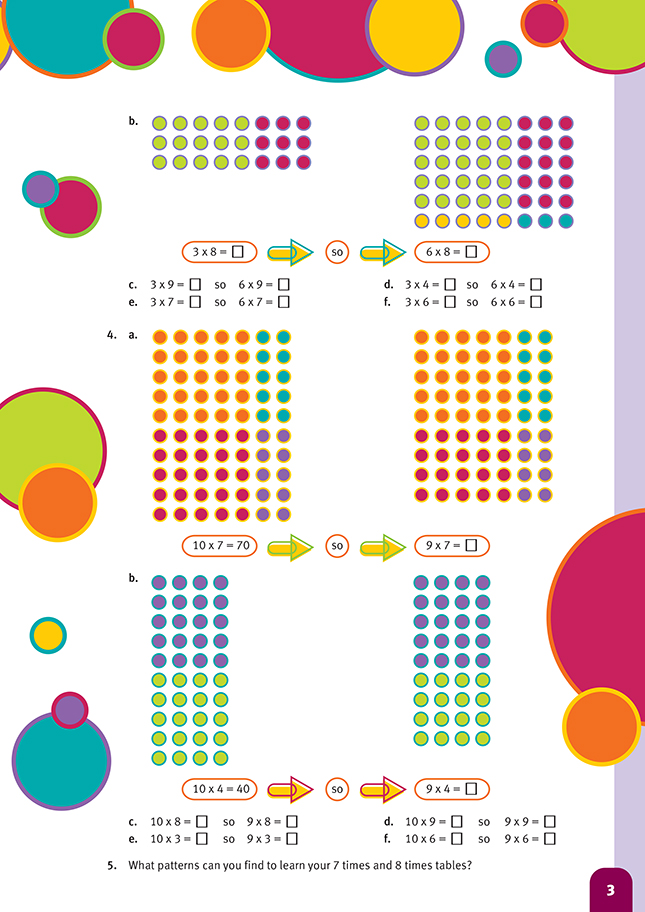



Table Tricks Nz Maths
You can put this solution on YOUR website! This item 3 ft x 2 ft Stainless Steel Worktable with Upturned Edge and Low Worktop $ TRINITY EcoStorage 72 in x 24 in Stainless Steel NSF Kitchen Utility Table with Adjustable Bottom Shelf9 G(x) 31 1 H(x) X 3 BPlot The Three Function In One Graph CWhen X Approaches 3 (but X 3) Does The Value Of Each




A 2 Column Table With 7 Rows The First Column Is Labeled X With Entries Negative 3 Negative 2 Brainly Com
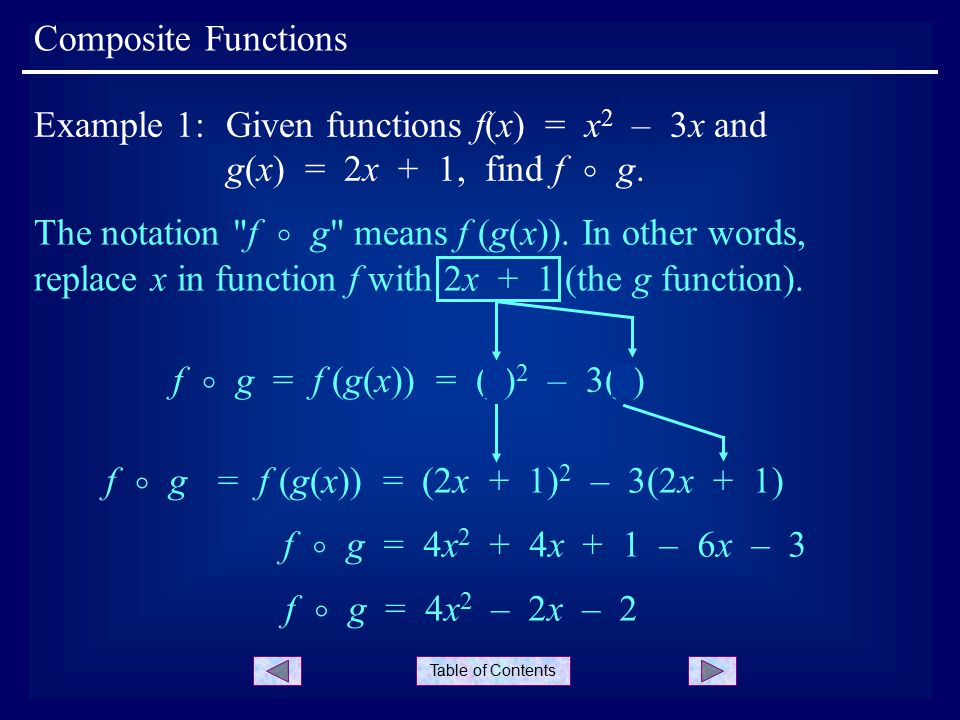



Table Of Contents Composite Functions Example 1 Given Functions F X X 2 3x And G X 2x 1 Find F G The Notation F G Means F G X In Ppt Download
X23(x4−1) View solution steps Short Solution Steps f ( x ) = 3 x ^ { 2 } \frac { 3 } { x ^ { 2 } } f ( x) = 3 x 2 − x 2 3 To add or subtract expressions, expand them to make their denominators the same Multiply 3x^ {2} times \frac {x^ {2}} {x^ {2}} Click here 👆 to get an answer to your question ️ the exponential function modeled by the following table?But let's use "f" We say "f of x equals x squared" what goes into the function is put inside parentheses after the name of the function So f(x) shows us the function is called "f", and "x" goes in And we usually see what a function does with the input f(x) = x 2 shows us that function "f" takes "x" and squares it




Graphing F X X 2 Using A Table Of Values Youtube



In Chapters 2 And 3 You Studied Linear Functions Of The Form F X Mx B A Quadratic Function Is A Function That Can Be Written In The Form Of F X
So, how can we write a function that's 0 at those places?Office Star Resin 3Piece Folding Bench and Table Set, 2 Benches and 6 x 25Feet Table, White,3 Piece Rectangle Table and Benches 47 out of 5 stars 1,997 $ $ 173 52This may be phrased with the equation lim x → 2 (3 x 5) = 11, lim x → 2 (3 x 5) = 11, which means that as x x nears 2 (but is not exactly 2), the output of the function f (x) = 3 x 5 f (x) = 3 x 5 gets as close as we want to 3 (2) 5, 3 (2) 5, or 11, which is the limit L, L, as we take values of x x sufficiently near 2 but not at



Http Www Mayfieldschools Org Downloads 1 1 warm up after lesson key Pdf
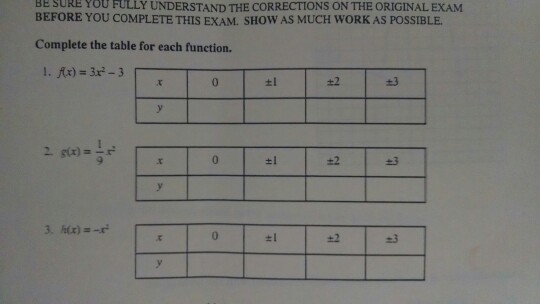



Complete The Table For Each Function F X 3x 2 Chegg Com
For these solutions to exist, the discriminant should not be a negative number First, we need to compute the discriminant Discriminant d=0 isAmazon Brand – Rivet Industrial Coffee Table with Multisquare Wood Design, 4724"W 47 out of 5 stars 4 $ $281 11 VINGLI 3FT Aluminum Folding Table,354''L x 236''W for Picnic Camping Party BBQ, Portable Utility Outdoor Tables with Carrying Handle 42 out of 5 starsSubscribe for new videos wwwyoutubecom/channel/UCIWCSw8jNs9SPetsVPo1WQQShare this video https//youtube/qpnJcI6XuQMThe problem Make a function table fo



Www Shsu Edu Kws006 Precalculus 1 1 Function Definition Files Ws Soln 1 1a Functiondefinition Pdf



2 Times Table Worksheet Worksheet English German Englisch
If now you wish to plot y = f(x) 2 then, for each x value, use the table above to finf f(x) and then add 2 to get f(x) 2 For example when x = 4 then f(x) = 0 so f(x) 2 = 0 2 = 2 and x = 3 then f(x) = 2 so f(x) 2 = 2 2 = 0 Thus I getAlgebra Examples Popular Problems Algebra Find the Inverse Function f (x)=2x3 3 f(x) 0 1 1 3 2 7 3 13 4 21 3 Plot the following table of values on the cartesian plane;




Need Help Create A Table Of Points From The Equation F X 3x 2 8x 2 Brainly Com



2fv5d843v9w22sxtto1ibxtu Wpengine Netdna Ssl Com Wp Content Uploads 15 11 Algebra F Straight Line Graphs V3 Solutions 1 Pdf
Question 1 Calculate the average rate of change of the function f(x) = x2 2x between x = 0 and =3 21 2 Use the following table of values to approximate the instantaneous rate of change of f(x) at x = 2 Explain why you chose the points that you In this math video lesson I review how to graph the exponential equation y=2^x by making a table The table helps when graphing these types of equations #eThose exact places, at x= 1, 2, 3, and 4 (13) Lee Ah If we write a function that's zero at x= 1, 2, 3, and 4 and add that to our f, the resulting function will have the same values as f at x= 1, 2, 3, and 4 That's the g we're looking for!



2
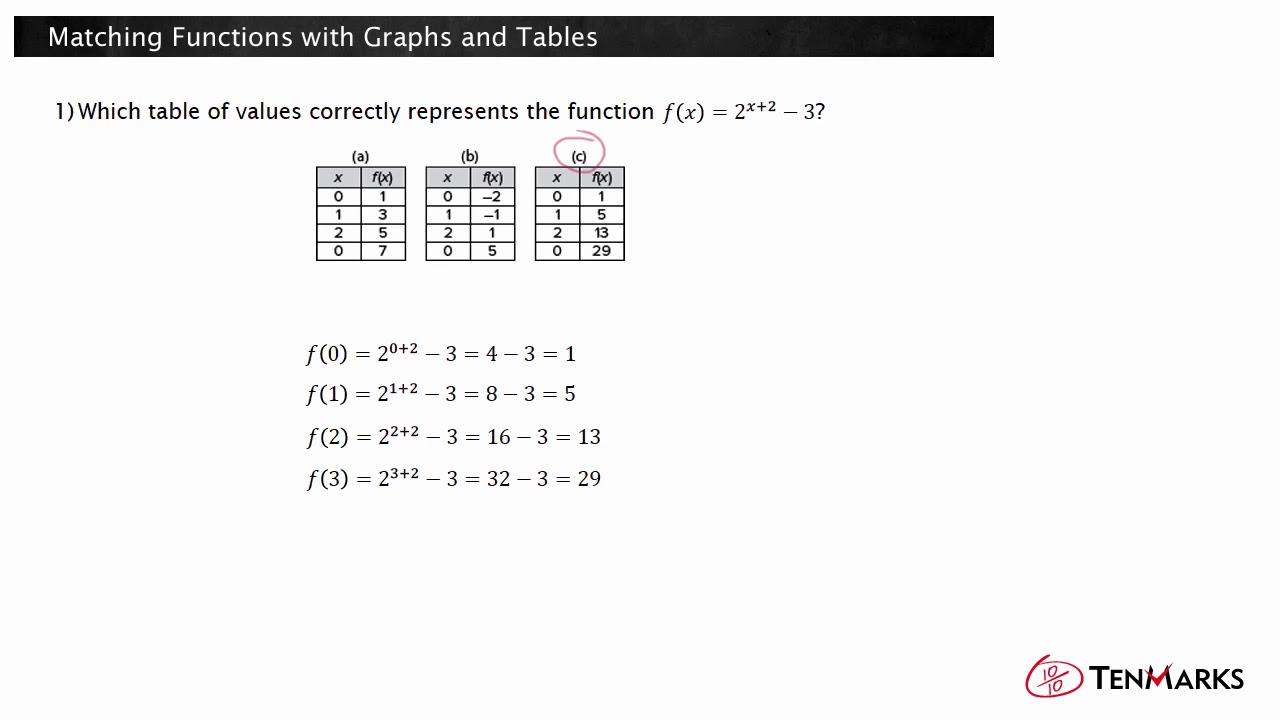



Matching Functions With Graphs And Tables F Le 3 Youtube



Search Q Y 3d3x Graph Tbm Isch



1



Math Scene Equations Iii Lesson 3 Quadratic Equations




Graph The Linear Equation Yx 2 1 Draw
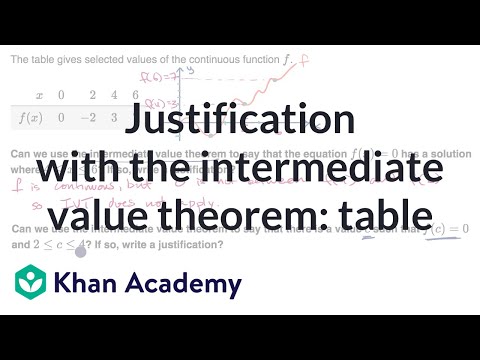



Justification With The Intermediate Value Theorem Table Video Khan Academy



Math Scene Equations Iii Lesson 3 Quadratic Equations



1
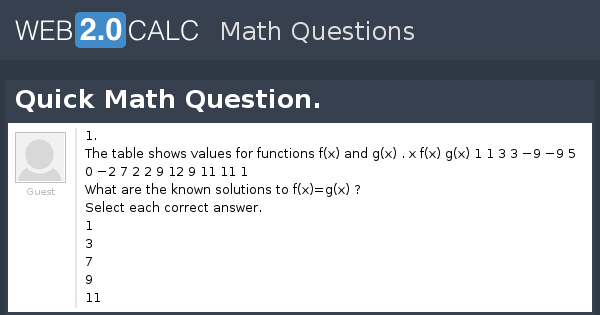



View Question Quick Math Question
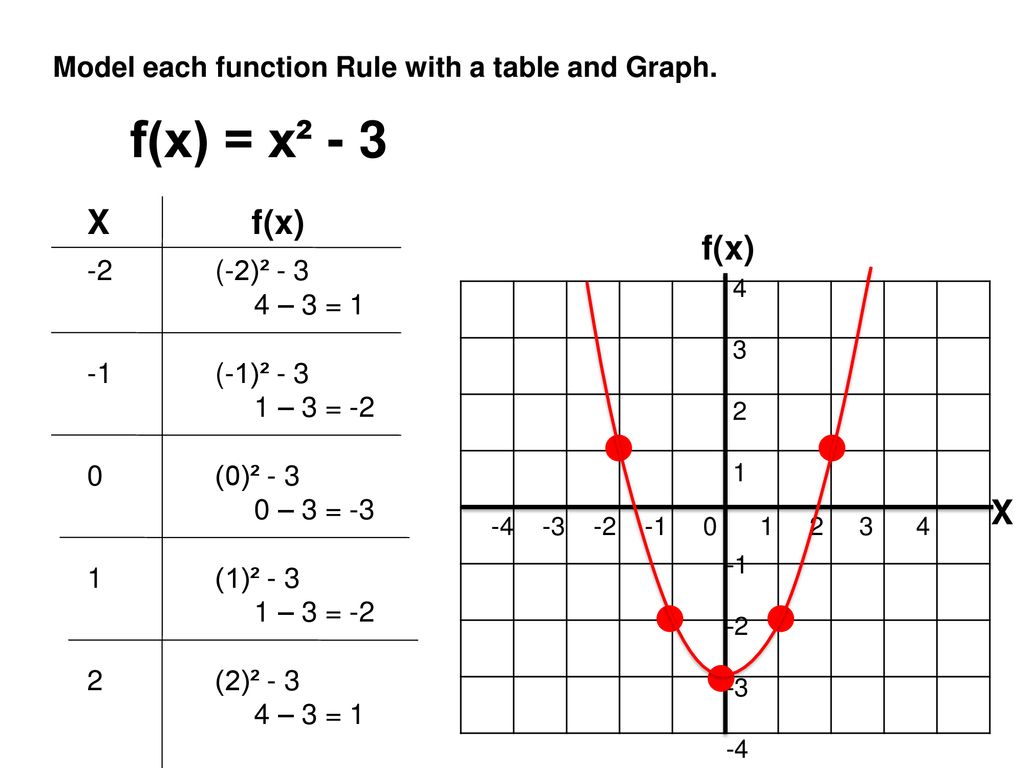



4 16 3 5 2 13 3 4 1 12 3 2 9 2 7 Ppt Download
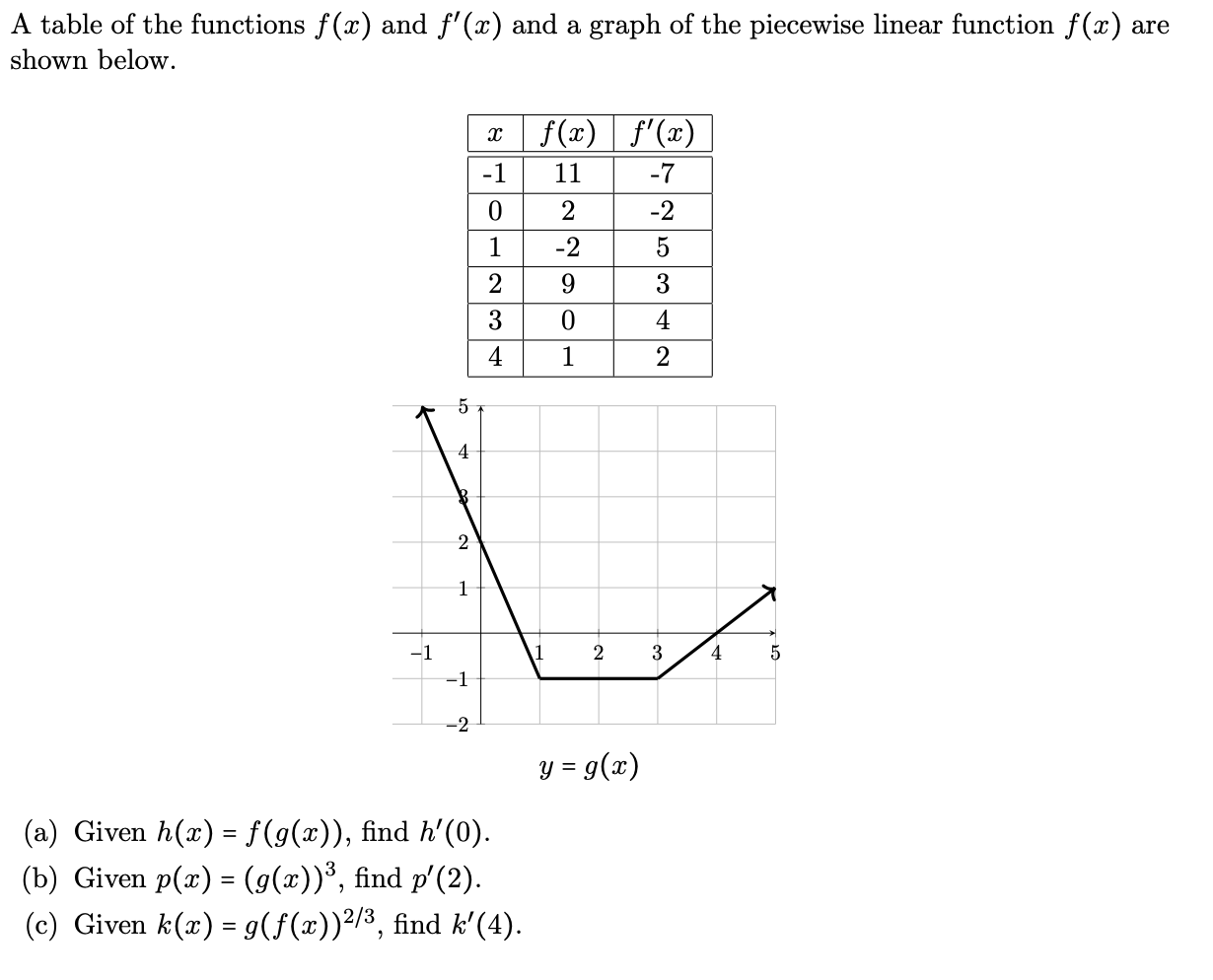



A Table Of The Functions F X And F X And A Graph Chegg Com




Here Is A Table Of Values For Y F X Mark The Statements That Are Truea The Domain For F X Is The Brainly Com




3 X Table Spaceright Europe Ltd



2




Find The Following Using The Table Below 2 1 F X 3 2 2 Homeworklib




2 2 The Graph Of A Function Mathematics Libretexts




Complete The Table For The Exponential Function F X 3 X 2 And Then Graph The Function Also Identify The Y Intercept For The Function Study Com



2



Http Www Mychandlerschools Org Cms Lib6 Az Centricity Domain 25 Module 10 review key Pdf



Www3 Nd Edu Apilking Math Work Old exams Exam2s17solutions Pdf




Answered Find The Following Using The Table Bartleby



2




Answered A Table Of Values Is Given For A Bartleby
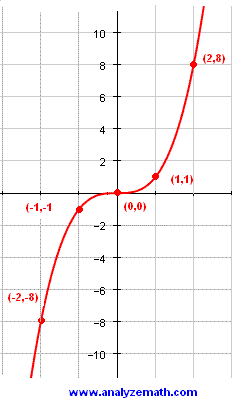



Graphing Cubic Functions
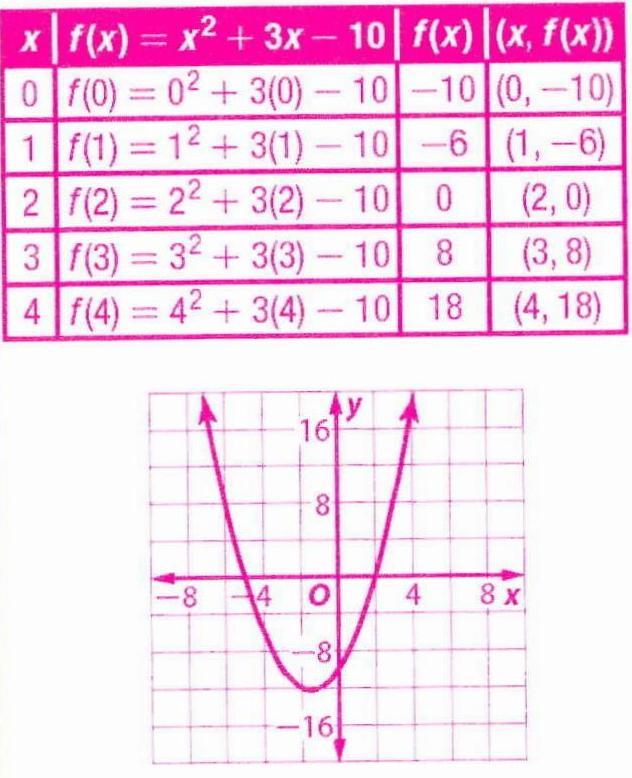



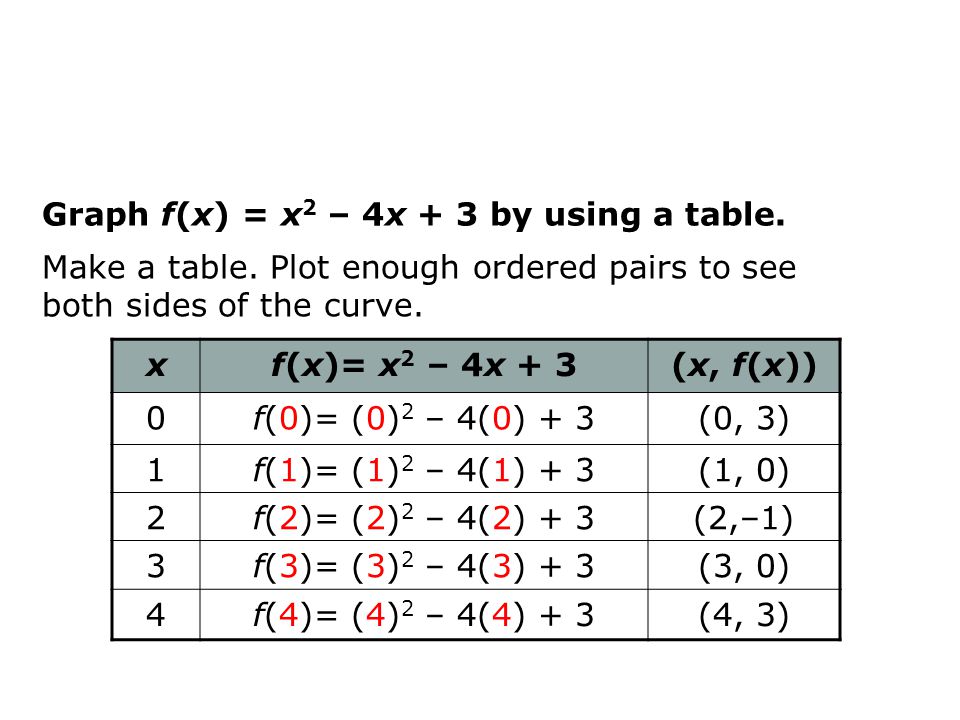
Graph F X X 2 3x 10 By Making A Table Of Values Snapsolve
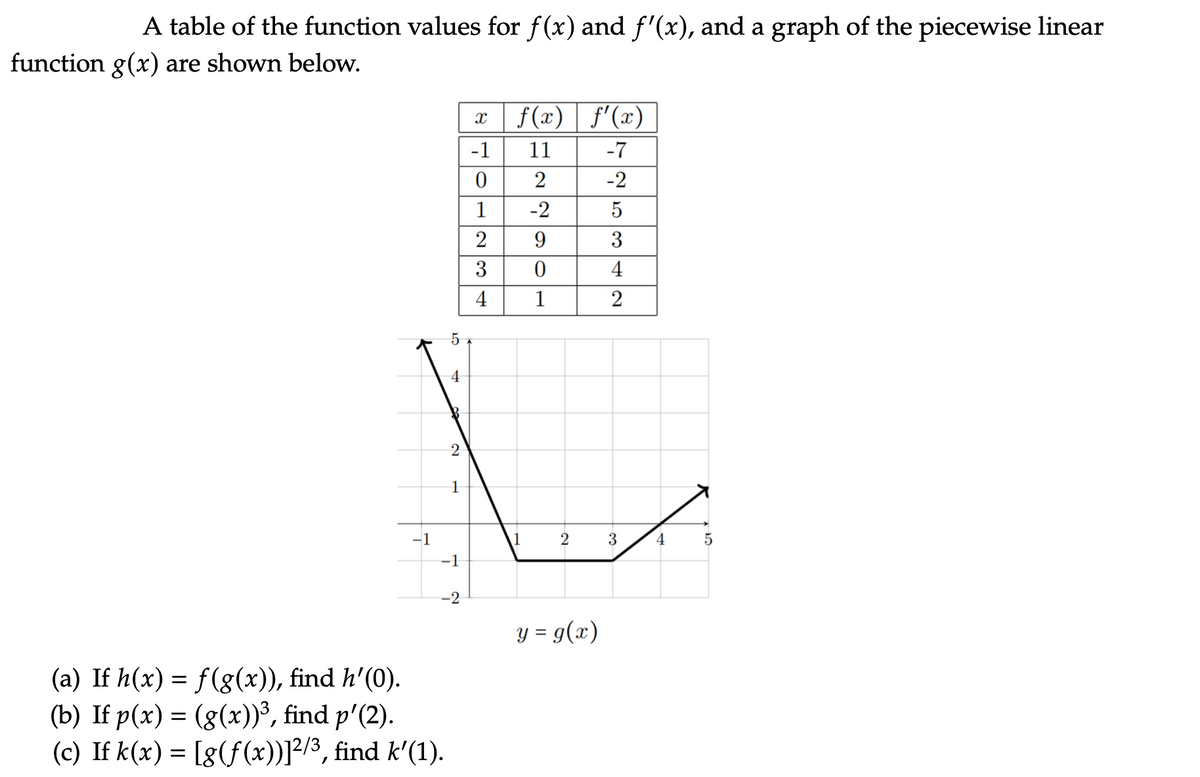



Answered A Table Of The Function Values For F X Bartleby



View Question Complete The Table For Each Function




1 Point Match The Functions Below With Their Level Surfaces At Height 3 In The Table At The Right 1 F X Y Z 22 3x 2 F X Y Z 2y 3x 3 F X Y Z 2y 3z 2




Ex 2 3 5 Find The Range Of Function F X 2 3x X R X 0



How Do You Graph F X X 2 X 5 4 And Identify The X Intercepts Vertex Socratic
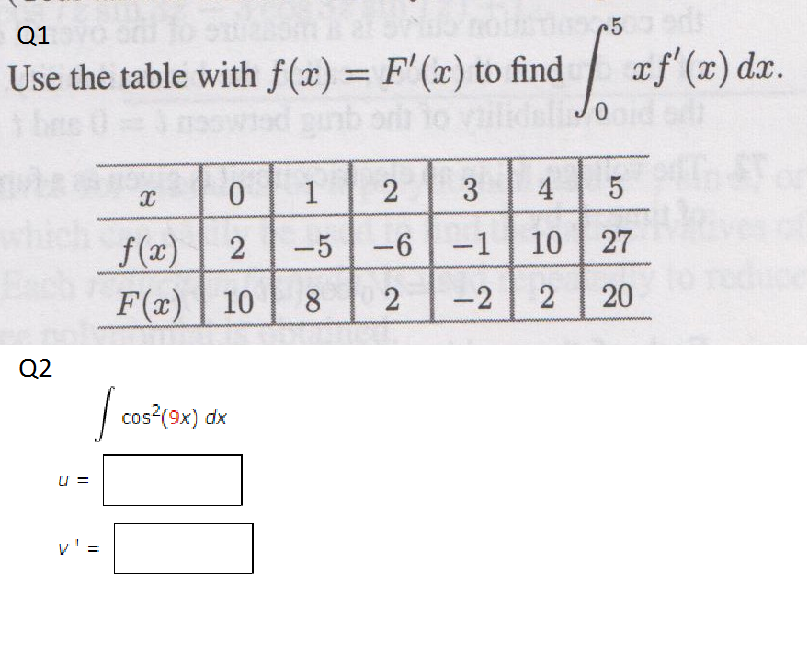



Q1 Use The Table With F X F X To Find X 0 1 2 Chegg Com




Given Z F X Y X X U V Y Y U V With X 5 2 3 Y 5 2 1 Calculate Z U 5 2 In Terms Of Some Of The Values Given In The Table Below F X 5 2 A F Y 5 2 2 X U 5 2



Function Tables




The Tables Below Show The Values Of F X And G X For Different V



Tables Of Piping Standards Size A B C D F K W Lt Itk Of Bars Silt O Bolts 2 41 2z 4 T 1 18 3 8 It I X Z 2
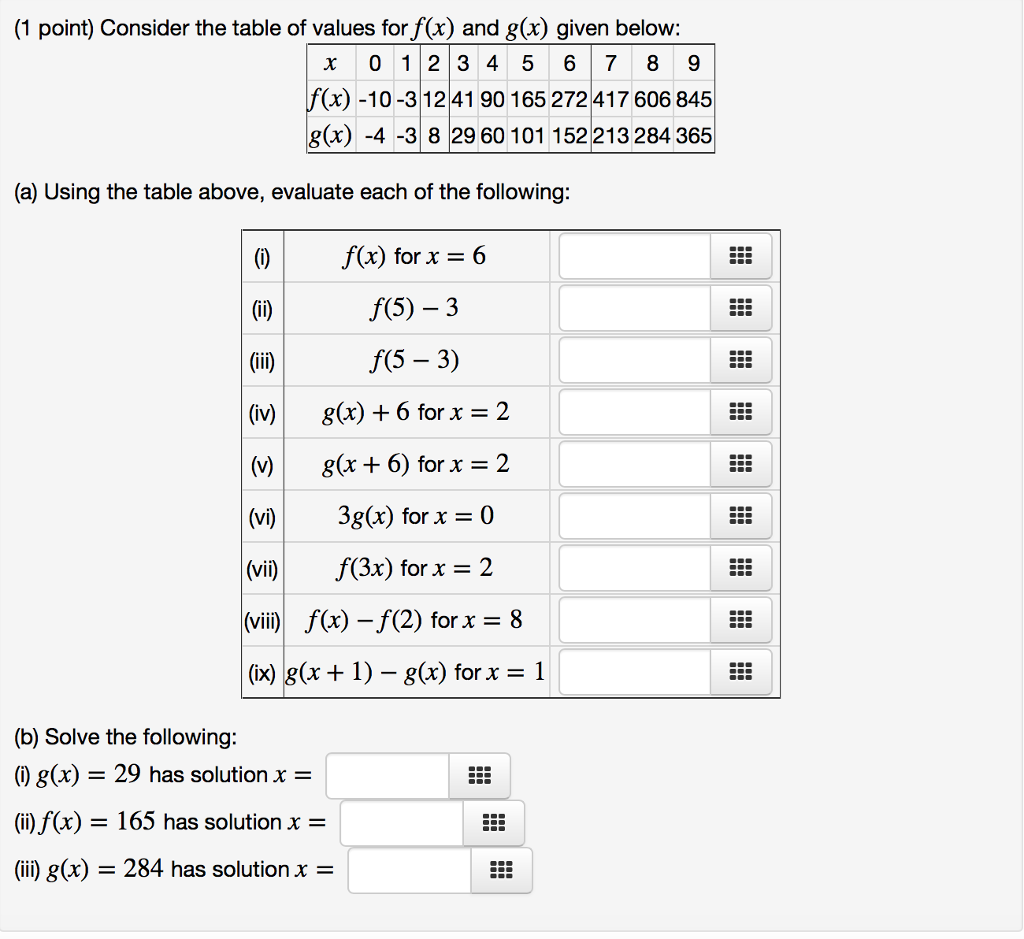



1 Point Consider The Table Of Values For F X And Chegg Com




Algebra 2 9 15 Intro To Quadratics




25pts Awarded And Brainliest Awarded Plz Help Asap Here Is A Table Of Values For Y F X X 2 Brainly Com



2




Help Needed With Calculus Question Wyzant Ask An Expert




Calculations Of Th Method For F X X 2 3x 2 0 A 2 Download Table




Find The Cubic Polynomial Which Takes The Following Values X 0 1 2 3 F X 1 2 1 10 M3 Notes Question Answer Collection




Use The Table To Evaluate Each Expression X 1 2 3 Chegg Com



2




Sketch The Graph Of The Exponential Function F X 3x Complete The Table Of Coordinates X Homeworklib
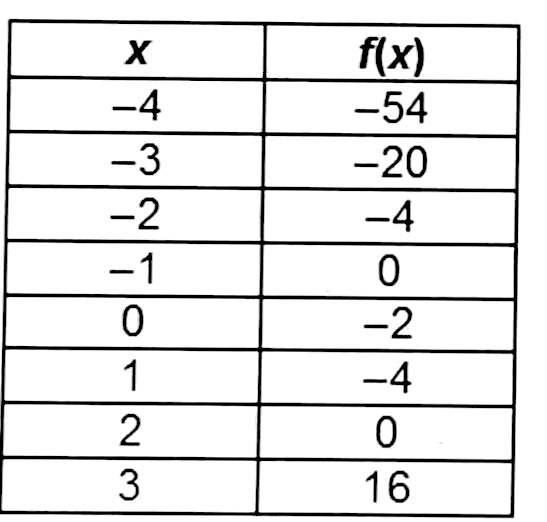



Using Only The Values Given In The Table For The Function F X X 3 3x 2 What Is The Interval Of X Values Over Which The Function Is Decreasing A 4 1 B 4 1 C 1 1 D 1 2 Snapsolve



Quadratic Functions And Inequalities 1 2 3 Graph




Ged Math Evaluating Functions Magoosh Ged Blog
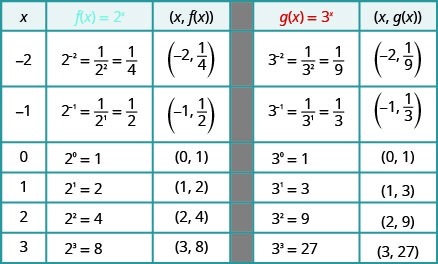



3 Evaluate And Graph Exponential Functions Mathematics Libretexts
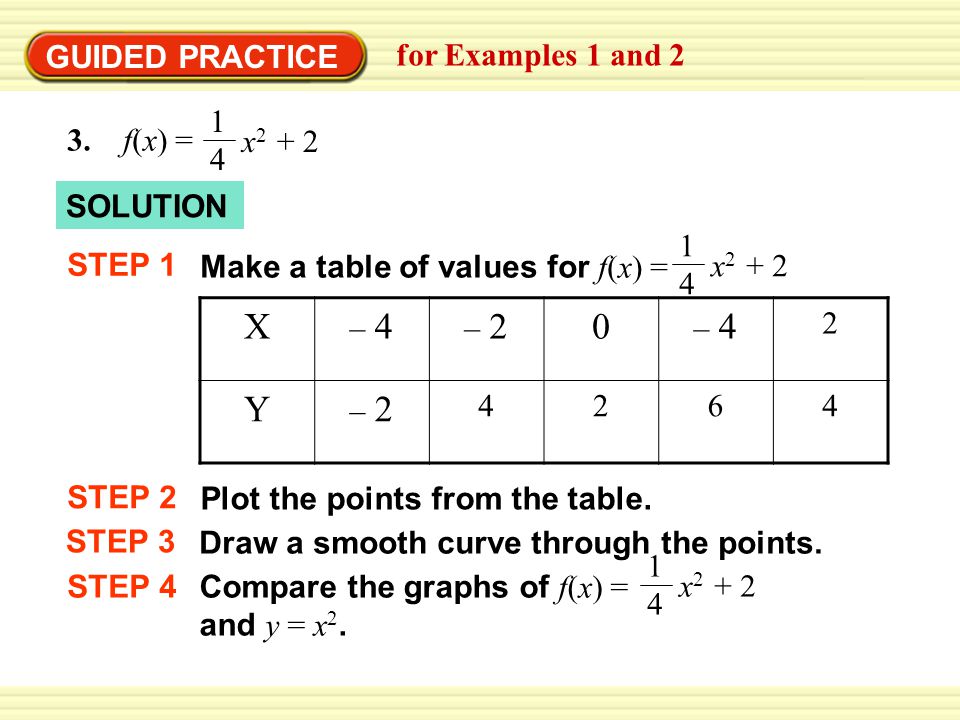



Example 1 Graph A Function Of The Form Y Ax 2 Graph Y 2x 2 Compare The Graph With The Graph Of Y X 2 Solution Step 1 Make A Table Of Values For Ppt Download




Estimating Derivative And Integral From Data Table Mathematics Stack Exchange



Help Me With This Construct A Table Of Values For The Function F X X 1 Is The Restricted Domain 0 0 5 1 1 5 2 2 5 3 Is This A One To One Function If It Is A One



Www Northernpolarbears Com Cms Lib Pa Centricity Domain 1107 Key chapter 1 part 1 Pdf




The Graph Of A Derivative F X Is Shown In The Figure Below Fill In The Table With Values For F X Given That F 0 8 Begin Array L L L L L L L L Hline X 0 1
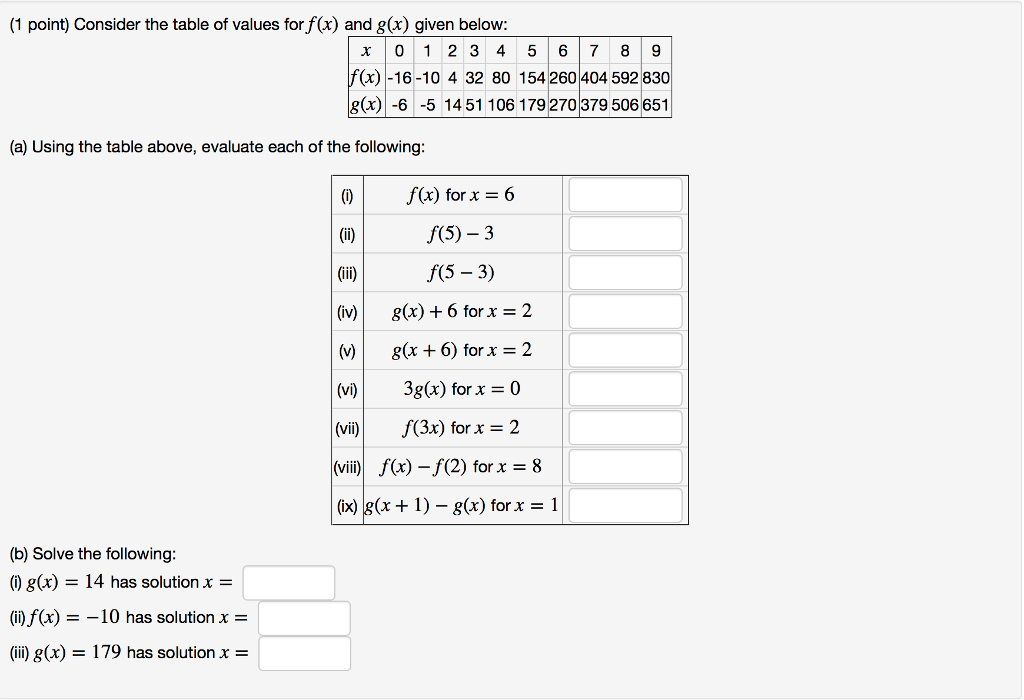



Consider The Table Of Values For F X And G X Chegg Com



Solving Equations Graphically




Solve Solve Inequalities With Step By Step Math Problem Solver



Http Www Humbleisd Net Cms Lib2 Tx Centricity Domain 3611 Answers inverse composition review0001 Pdf
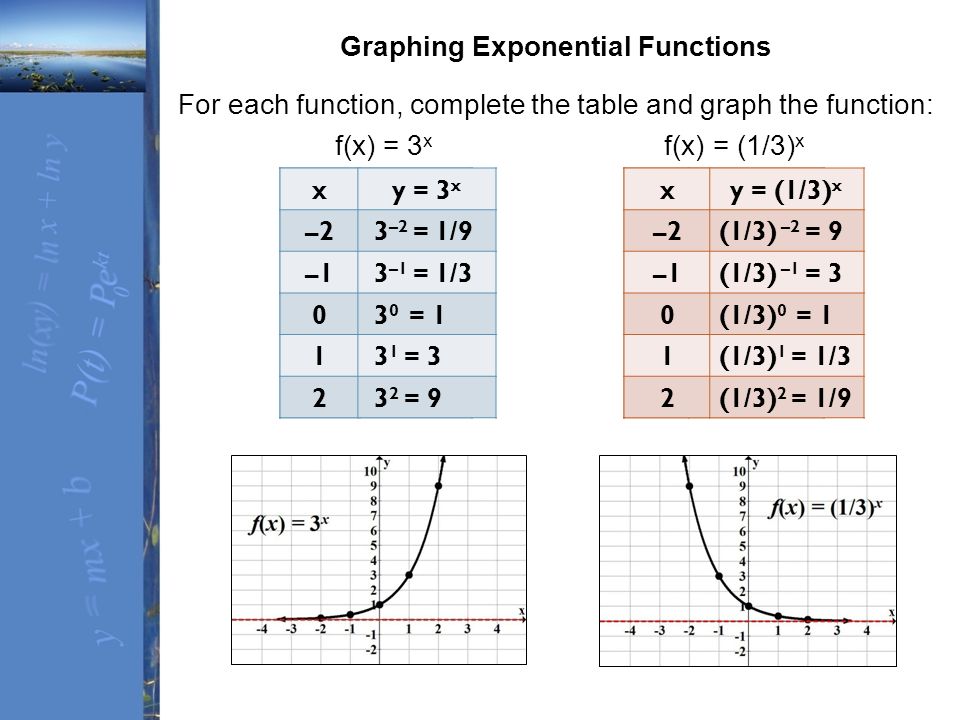



Chapter 6 Exponential And Logarithmic Functions And Applications Section Ppt Download



Ap Calculus Ab Semester Exam Review



Quadratics Graphing Parabolas Sparknotes



Quadratics Graphing Parabolas Sparknotes



Math Scene Inequalities Lesson 3 Absolute Values
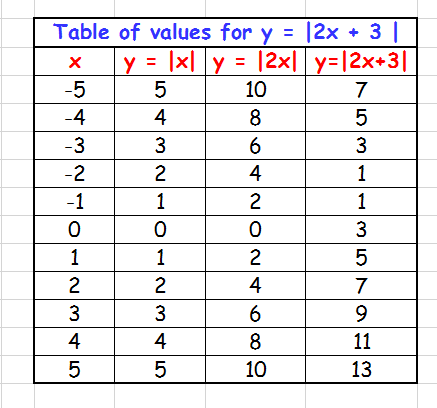



How Do You Graph F X Abs 2x 3 Socratic



Faculty Math Illinois Edu Lfolwa2 E2rubric Pdf



Using Only The Values Given In The Table For The Function F X X3 3x 2 What Is The Interval Of X Values Over Which The F




Ex Complete A Table Of Values Given A Function Rule Youtube
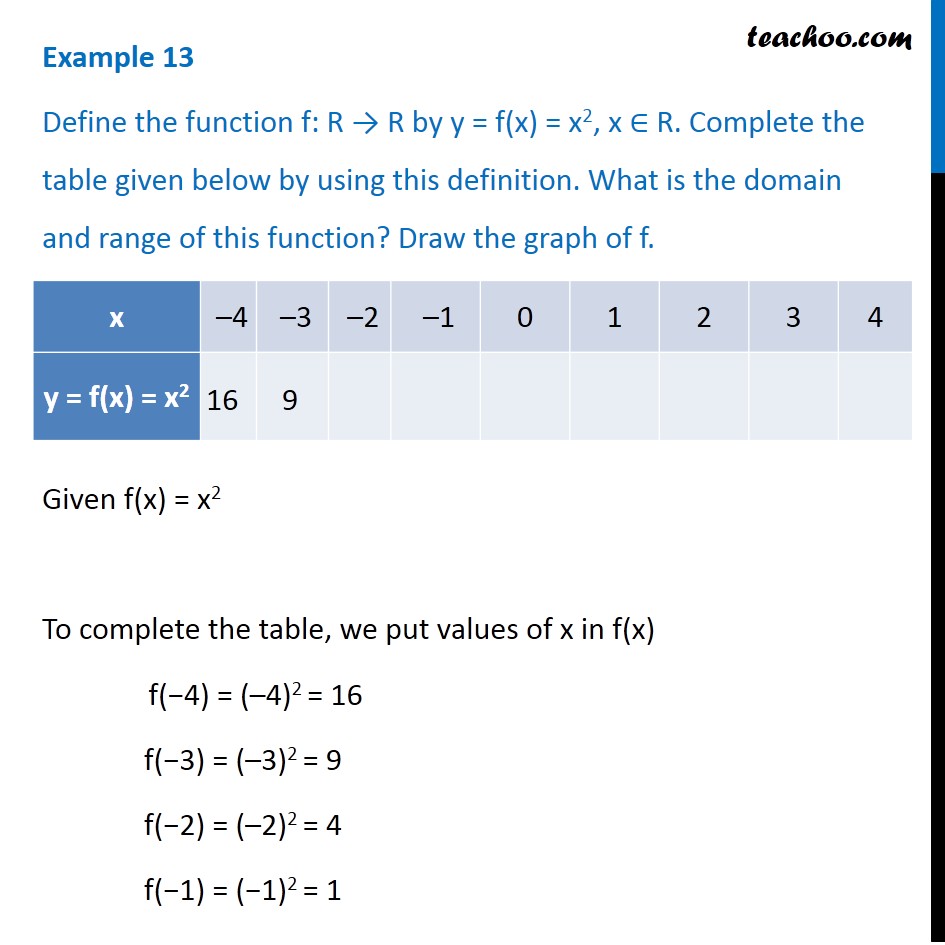



Example 13 Define Function Y F X X 2 Complete The Table




Abstract Interpolation Computational Science




How To Find The Range Of A Function Video Khan Academy



Tables Of Piping Standards A P P Uj U Ii I R Wa L Size A B C D F K W Lt Itk Of Bars Silt O Bolts 2 41 2z 4 T



Search Q Y 3d 3x 1 Table Tbm Isch




Consider The Function F X 3x 2 And The Function G X Which Is Represented By The Table Below Brainly Com




A Table Representing The Function F X 2 3 2 X Is Shown Below What Is True Of The Given Function Brainly Com



Cckefys Gdfqmm
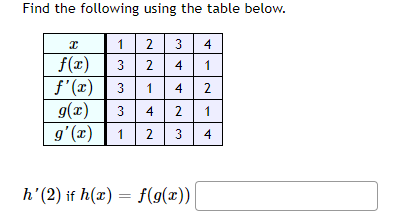



Answered Find The Following Using The Table Bartleby



Www Manhassetschools Org Cms Lib Ny Centricity Domain 217 11h cw 74 77 answer key Pdf



0 件のコメント:
コメントを投稿